Page 38 - Tequio 11

36 On some polytopes in phylogenetics/Hoessly/27-40

Then the form of Lipschitz polytopes of finite tree-like spaces can be given as follows.

Theorem 3.6 (Delucchi & Hoessly, 2020, Theorem 3.1) Let (, ) be a tree-like pseudometric space. Then,
(, ) = ∑ ã ã where (, ) is the unique weighted system of compatible splits of such that = ä
ã∈
(cf. Theorem 2.6).

We next go through an example.

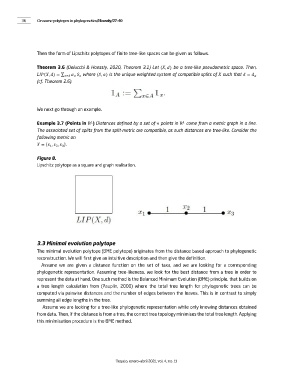
Example 3.7 (Points in ℝ ) Distances defined by a set of points in ℝ come from a metric graph in a line.
-
-
The associated set of splits from the split-metric are compatible, as such distances are tree-like. Consider the
following metric on
= { - , * , A }.

Figure 8.
Lipschitz polytope as a square and graph realisation.

3.3 Minimal evolution polytope

The minimal evolution polytope (BME polytope) originates from the distance based approach to phylogenetic
reconstruction. We will first give an intuitive description and then give the definition.
Assume we are given a distance function on the set of taxa, and we are looking for a corresponding
phylogenetic representation. Assuming tree-likeness, we look for the best distance from a tree in order to
represent the data at hand. One such method is the Balanced Minimum Evolution (BME) principle, that builds on
a tree length calculation from (Pauplin, 2000) where the total tree length for phylogenetic trees can be
computed via pairwise distances and the number of edges between the leaves. This is in contrast to simply
summing all edge lengths in the tree.
Assume we are looking for a tree-like phylogenetic representation while only knowing distances obtained
from data. Then, if the distance is from a tree, the correct tree topology minimises the total tree length. Applying
this minimisation procedure is the BME method.

Tequio, enero-abril 2021, vol. 4, no. 11