Page 33 - Tequio 11

On some polytopes in phylogenetics/Hoessly/27-40 31

Definition 2.4 (Tree-like metrics) A (pseudo)metric on a set is called a tree-like (pseudo)metric if there
exists an -tree (, ) and a weighting of such that for all , ∈

(, ) = w ((), ()).

The pseudometric is a metric if and only if is injective.

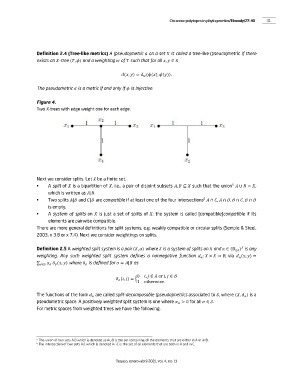
Figure 4.
Two X-trees with edge weight one for each edge.

Next we consider splits. Let be a finite set.
• A split of is a bipartition of , i.e., a pair of disjoint subsets , ⊆ such that the union ∪ = ,
5
which is written as |.
• Two splits | and | are compatible if at least one of the four intersections ∩ , ∩ , ∩ , ∩
6
is empty.
• A system of splits on is just a set of splits of ; the system is called [compatible]compatible if its
elements are pairwise compatible.
There are more general definitions for split systems, e.g. weakly compatible or circular splits (Semple & Steel,
2003, x 3.8 or x 7.4). Next we consider weightings on splits.

Definition 2.5 A weighted split system is a pair (, ) where is a system of splits on and ∈ (ℝ n, ) is any

weighting. Any such weighted split system defines a nonnegative function ä : × → ℝ via ä (, ) =
∑ ã∈ ã ã (, ) where ã is defined for = | as

0 , ∈ or , ∈
ã (, ) = é
1 otherwise.

The functions of the form ä are called split-decomposable (pseudo)metrics associated to , where (, ä ) is a
pseudometric space. A positively weighted split system is one where ã > 0 for all ∈ .
For metric spaces from weighted trees we have the following.

5 The union of two sets A,B which is denoted as A∪B is the set containing all the elements that are either in A or in B.
5
The union of two sets , which is denoted as ∪ is the set containing all the elements that are either in or in .
6 The intersection of two sets A,C which is denoted A∩C is the set of all elements that are both in A and in C.
6 The intersection of two sets , which is denoted ∩ is the set of all elements that are both in and in .
Tequio, enero-abril 2021, vol. 4, no. 11