Page 34 - Tequio 11

32 On some polytopes in phylogenetics/Hoessly/27-40

Theorem 2.6 ((Semple & Steel, 2003, Theorems 3.1.4, 7.1.8, 7.3.2)) Let (, ) be a pseudometric space.
The following are equivalent:
(i) is a tree-like pseudo-metric on (in the sense of Definition [df:tm2]).
(ii) is a split-decomposable pseudometric associated to a positively weighted system of compatible splits.
Moreover, this system is unique.
7
Under the equivalence of (I) with (II), splits in the decomposition of the metric correspond bijectively to edges
in the tree.

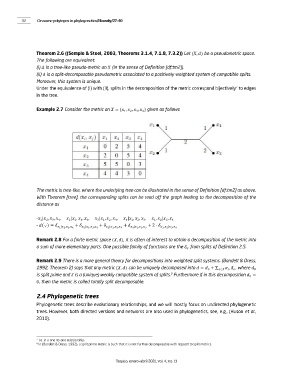
Example 2.7 Consider the metric on = { - , * , A , ë } given as follows

The metric is tree-like, where the underlying tree can be illustrated in the sense of Definition [df:tm2] as above.
With Theorem [tree], the corresponding splits can be read off the graph leading to the decomposition of the
distance as

- - | * , A , ë , * | - , A , ë , A | - , * , ë , ë | - , * , A , - , * | A , ë

+ î 1 |î . ,î / ,î 0
+ 2 ⋅ î . ,î / |î 0 ,î 1
+ î / |î . ,î 0 ,î 1
- (⋅,⋅) = î . |î / ,î 0 ,î 1
+ î 0 |î . ,î / ,î 1

Remark 2.8 For a finite metric space (, ), it is often of interest to obtain a decomposition of the metric into
a sum of more elementary parts. One possible family of functions are the ã from splits of Definition 2.5.

Remark 2.9 There is a more general theory for decompositions into weighted split systems. (Bandelt & Dress,
1992, Theorem 2) says that any metric (, ) can be uniquely decomposed into = , + ∑ ã∈ ã ã , where ,
8
is split prime and is a (unique) weakly compatible system of splits. Furthermore if in this decomposition , =
0, then the metric is called totally split decomposable.

2.4 Phylogenetic trees

Phylogenetic trees describe evolutionary relationships, and we will mostly focus on undirected phylogenetic
trees. However, both directed versions and networks are also used in phylogenetics, see, e.g., (Huson et al.,
2010).

7 I.e. in a one-to-one relationship.
7
8 I.e. in a one-to-one relationship.
In (Bandelt & Dress, 1992), a split prime metric is such that it is not further decomposable with respect to split metrics.
8 In (Bandelt & Dress, 1992), a split prime metric is such that it is not further decomposable with respect to split metrics.
Tequio, enero-abril 2021, vol. 4, no. 11